Introduction

The CVA risk measure is actually a group of risk measures (CVA, DVA, and Bilateral CVA).

Credit valuation adjustment (CVA) is the cost of buying protection that pays the portfolio value in the case of the counterparty defaulting, while debit valuation adjustment (DVA) is the cost for a counterparty to buy protection that pays the portfolio value in the case of the institution defaulting.  For CVA, it is assumed the default probability of the institution is zero while for DVA, it is assumed the counterparty does not default.  In the case of bilateral CVA, both the institution and counterparty can default.  In the Vector Risk Engine we assume the default of the institution and the counterparty are independent but they cannot both default simultaneously.

The CVA calculation in the Vector Risk Engine is described by the equation:




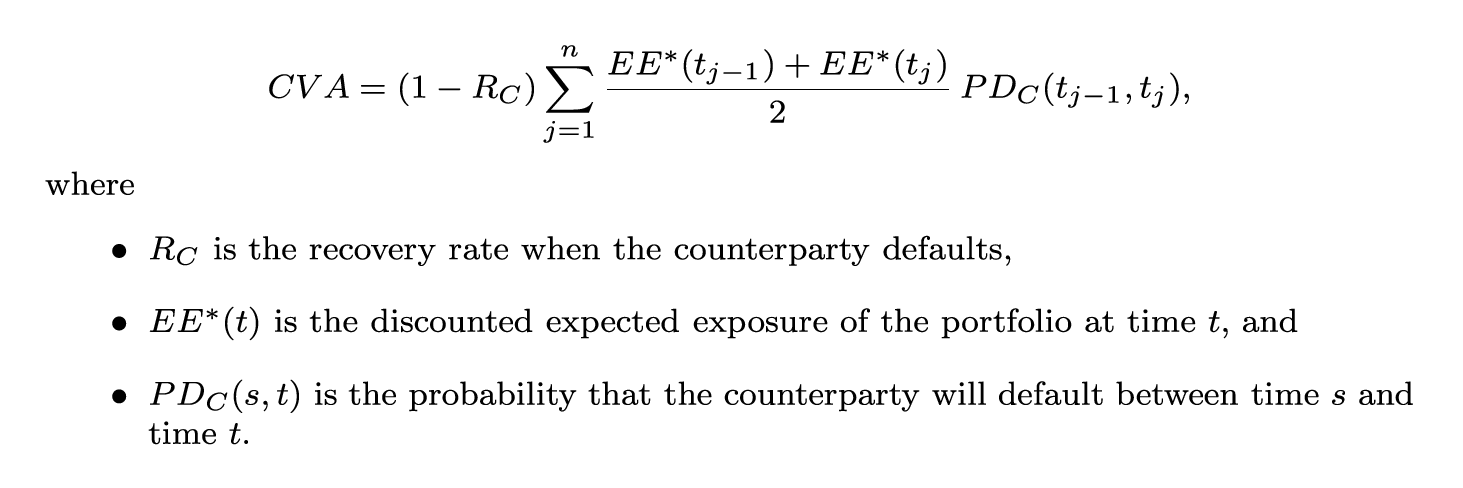
The DVA calculation is described by the equation:





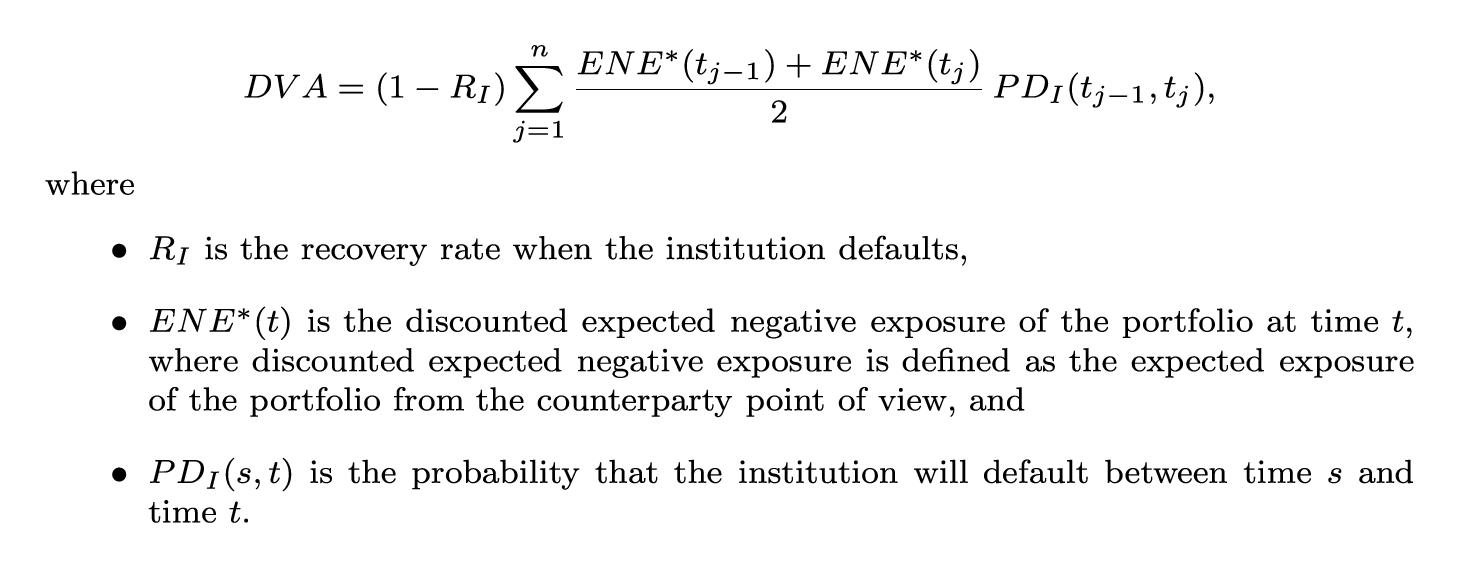
The Bilateral CVA calculation is described by the equation:




Risk Neutral Processes

CVA is a market-based measure of the price of counterparty risk and as such is generally calculated using a risk neutral framework with accompanying risk neutral stochastic processes. CVA trading and hedging is possible and so it should be calculated with this in mind. This differs from the more standard risk measures such as potential future exposure where real world scenarios are used as there is no traded market for these measures.

Wrong Way Risk

In the standard CVA formula, it is assumed the expected exposure and default probably are independent.  However, this assumption is not necessary realistic.  ISDA states that “wrong way risk occurs when exposure to a counterparty is adversely correlated with the credit quality of the counterparty”.  In Vector Risk risk engine, wrong way risk is captured by calculating default probability of each scenario of the simulation.

Margin Period of Risk

The measurement of CVA on collateralized portfolios must account for the margin period of risk (MPoR) that recognizes the potential timing mismatch between calling for collateral and closeout of positions.

In fact we comply fully with the Basel document "Review of the Credit Valuation Adjustment Risk Framework" which states:

"For transactions that are covered by a legally enforceable margin agreement, the simulation must capture the effects of margining collateral along each exposure path. All the relevant contractual features such as the nature of the margin agreement (unilateral vs bilateral), the frequency of margin calls, the type of collateral, thresholds, independent amounts, initial margins and minimum transfer amounts must be appropriately captured by the exposure model. To determine collateral available to a bank at a given exposure measurement time point, the exposure model must assume that the counterparty will not post or return any collateral within a certain time period immediately prior to that time point. The assumed value of this time period, known as the margin period of risk (MPoR), cannot be less than a supervisory floor."